3. ELLIPSOIDS: BULGES, ELLIPTICAL GALAXIES, AND GLOBULAR CLUSTERS

3.1 Surface Photometry

• Review of techniques (photographic,CDD)
  
• Radial brightness profiles:
  
  • Systematics
    
  • Analytic fitting functions
    
• D galaxies: properties of cD halos
  
• Cores: ground-based and HST observations
  
  

3.2 Families of Ellipsoidal Stellar Systems

• Global and core parameter correlations
  
• Three kinds of stellar systems: bulges + ellipticals, Sphs, globulars
  
• Relationship between Sph and S + I galaxies: Introduction
  
  

3.3 The Fundamental Plane of Elliptical Galaxies

• Global and core parameter correlations
  
• Scalar virial theorem; derivation of the fundamental plane equations
  
• Implications for galaxy formation; M/L (L )
  
  

3.4 Stellar Dynamics:Observations

• Measurement techniques:
  
  • cross-correlation,Fourier quotient,Fourier correlation quotient,...
    
• Line-of-sight velocity distributions (LOSVDs)
  
• Observations of rotation and velocity dispersion pro •les
  
• Velocity anisotropy: the diagram
  
• Observational confirmation of triaxiality and anisotropy:
  
  • Minor-axis rotation
    
  • Shapes of E galaxies:statistics of apparent shapes
    
  • Isophote twists
    
  • Dust rings:implications for E shapes
    
    

3.5 Isophote Shapes:Boxy and Disky Es

• Measurements of isophote shapes: a (4)/a
  
• Correlations of a (4)/a with physical properties
  
• Physical dichotomy of E galaxies into
  
  • (1)High-L , nonrotating, boxy, anisotropic Es with cuspy cores, and
    
  • (2)Medium-and low-L , rotating, disky, approximately isotropic and coreless Es
    
• Proposed revision of the Hubble sequence: boxy E –disky E –S0 –Sa –Sb –Sc –...–Im
  
• Exceptions: Boxy bulges and low-L boxy Es: origin
  
  

3.6 Collisionless Dynamics: Theory

• Stellar systems are fundamentally more complicated than gases:
  
  • Long mean free path
    
• Characteristic times: crossing time; relaxation time
  
• Distribution function
  
• Fundamental equations of macroscopic stellar dynamics:
  
  • Collisionless Boltzmann equation
    
  • Poisson equation
    
• First moment equations =basic equations of stellar hydrodynamics:
  
  • The Jeans equations
    
• Second moment equations: tensor virial theorem
  
• Application to diagram ==> anisotropy
  
  

3.7 Galaxy Models. I. f =f (E, L_z )

• Jeans Theorem
  
• Models with f =f (E ):polytropes,isothermals,King models
  
  • Emphasize:similarities between stellar dynamical and gas case (i.e.,stars)
    
  • 
    
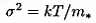
  • Core mass-to-light ratios
    
• Models with f =f (E,L_z )
  
  

3.8 Globular Cluster Observations and Models

• Density distributions
  
• Velocity dispersion profiles
  
• Models with a range of stellar masses m
  
  

3.9 Stellar Orbits in Ellipsoidal Stellar Systems

• Orbits in a spherical potential
  
• Orbits in axisymmetric potentials: classical integrals
  
• Orbits in triaxial potentials
  
  

3.10 Galaxy Models.II.Galaxy = weight_i orbit_i

• Schwarzschild ’s method; examples
  
• Spherical maximum entropy models; examples
  
• Axisymmetric maximum entropy models; examples
  

3.11 Dynamical Evolution of Ellipticals and Globular Clusters: Theory

• Phase mixing and violent relaxation
  
  • Origin of the 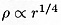density distribution
    
• Two-body encounters and relaxation
  
  • Heat capacity of a self-gravitating stellar system is negative
    
  • Core collapse: single- simulations
    
  • Stopping core collapse via binaries
    
  • Gravothermal oscillations
    
  • Complications: range of , primordial binaries, stellar evolution, physical stellar collisions, stellar coalescence, runaway stellar mergers,...
    
• External influences:
  
  • Tidal effects
    
  • Disruption of globular clusters by galactic disk shocking
    
  • Relation between present and primordial globular cluster population
    
    

3.12 Dynamical Evolution of Ellipticals and Globular Clusters: Observations

• Post-core-collapse density distributions in globulars
  
• Mass segregation
  
• Stellar population gradients:blue stragglers
  
• Stellar population gradients in bulges and Es <== effects of high stellar density
  
  

3.13 Supermassive Black Holes (BHs)in Galactic Nuclei

• Brief motivation: nucelar activity (see §9)
  
• Origin of seed BHs via evolution of dense stellar systems
  
• Stellar-dynamical search for BHs
  
• Gas-dynamical search for BHs
  
• BH demographics
  
• Flashes when stars are accreted by BHs